In this trilogy, the author takes us on a journey through the language of math, beginning with exploring five important equations that forever changed how we see the world, from the ancient Pythagorean theorem to quantum physics.
Through the language that is mathematics, some of the most gorgeous and powerful descriptions of our natural reality have been put forward by the best of us.
Before we begin...
When teachers plan a math lesson, especially one that begins a new topic, we often think: What’s a good opening problem? Ninety-nine percent of the time, this leads my mind right into physics. With physics, math becomes meaningful and rigorous. With physics, you get to come to grips with some of the most mind-boggling and awe-inspiring mathematical concepts. With physics, teachers like us get the chance to guide your understanding in a productive manner.
Through the language that is mathematics, and through the passage of time, as well as under the guidance of our innate curiosity and capacity for humility, some of the most gorgeous and powerful mathematical descriptions of our natural reality have since been put forward by the best of us—in our grand pursuit of a theory of everything. Though right now, we are not quite there yet, perhaps not even close. But this is actually a blessing in and of itself, for reality may very well turn out to be an incompletable puzzle after all. Still, year after year, and generation after generation, we inch ever closer to the entire picture. One piece at a time, and over thousands of years, the human species has since taken on the challenge to decode the entire universe, by using our best available tool, mathematics.
At the same time, however, as our physical and mathematical theorems grow in complexity and depth, we’re also getting impatient, and soon, skeptical. Think about this: Our greatest solution is probably also our greatest problem. Could our mathematics be wrong, or at least flawed? If so, to which extent? And might you one day rise to this ultimate challenge of bringing our understanding to the next level?
Naturally, that begins by taking a look at our history of discovery, learning not only from the celebrated moments, but also from what we had come to learn was misguided and, worse, outright wrong. On the one hand, we have gone from wheels to rockets in a matter of millennia, but on the other, and ever more so, in our present reality, unprecedented environmental destruction and climate change.
Now, with these in mind, and without further ado, let’s begin the first part of this essay by taking a look at, among many, the five equations that changed our view of the universe forever.
#1 : Probably not Pythagoras
It’s easy to brush off the Pythagorean theorem as being simple or obvious. If we are referring to ‘simple’ as being ‘I can solve exam questions with it as well as my calculator’, then that’s absolutely right.
But the ancient quest to compute the length of the hypotenuse of a right-angled triangle with a height and a base of each 1 unit in length, eventually led to the idea of, well, the square root of 2. Wait, what was the square root of 2? You tell me – we modern humans don’t usually think much about this problem, but when we do, it deepens our historical perspective and, in my view, makes us all better and more sensitive learners. Consider this: Nearly 4,000 years ago, the mathematical intuition of our species took a giant leap – from a universe governed by integers to the then-heresy we now simply take for granted, a.k.a. nonintegers. Maybe I’m a fanatic, but I think that it simply turned out great that we now have infinite values between 0 and 1. And let’s just say that this step in our mathematical history might just be bigger than Armstrong’s moonwalk.
#2 : The falling apple
So, speaking of the lunar landing back in 1969, how did we make it there – I mean, 384,400 kilometers, or roughly 32 Earths (yes, 32) away from us? To answer that, let’s talk about love. Whenever I get asked relationship advice (not that I actually do), particularly “Does long-distance relationship work?”, I inevitably bring Newton up to the conversation, which would, I imagine, turn awkward very quickly. Anyway, for better or worse, it would probably go like this:
“How far away will she be?”
“Well, I’ll stay here in KL, and she’s going to live in London.”
“I see. Look, she’ll be roughly 100 million times less attracted to you.”
I know what you’re thinking: gravity isn’t love (nonetheless, if you’re curious as to how that number came about, it only came from this equation and took about two and a half minutes to compute). But the idea that every object with mass exerts an attractive force on any other object across the entire universe, and vice versa; and the fact that some guy figured this out more than 300 years ago after an apple fell before him? One cannot simply deny that gravity is both mysterious and totally awesome. The universe, and all of its stuff – from the tiniest to the largest, obey it. Gravity rules the cosmos. It rules our galaxies and stars and planets and moons. It rules our calculations that made the Apollo 11 mission a triumph. And, here on Earth, it rules all of life.
Although Newton didn’t technically discover gravity (I firmly believe that our first ancestor did, and by that, I don’t mean humans or apes even), words cannot describe how immensely important this formula that he derived truly has been to us. For one thing, we have it to thank whenever we log on to the internet. Yes, that includes every silly text you (and I) sent and regretted. Then, merely consider the fact that this equation describes how everything is attracted to everything. That means, since the inception of this equation, we can now accurately utilize Earth’s gravitational field, and hence everything we have achieved and are working on up to this point in time – from Sputnik, Apollo 11, the ISS, all the way to our ongoing, absolutely wildest dreams of our establishing an interplanetary presence, beyond our blue-green little rock floating in space – has since become such a part of our collective consciousness, and will definitely continue to challenge and extend the way we see ourselves as we progress into the future.
Practically, though, the most profound contribution that Newton’s gravitational equation was that it gave us none other than G itself, the symbol of the universal gravitational constant. I mean, let this sink in for a moment: how on earth does a number such as 0.0000000000667 govern everything?
#3 : a matter of perspective
Once in a lifetime, a genius pops out in this otherwise mundane human realm we have been confined in, and occasionally—you know—sparks one or two scientific revolutions. The year was 1905. Summer had just ended in Bern, Switzerland. A 26-year-old patent office clerk, despite the banality, was on the brink of an unimaginably remarkable discovery. Here, let the genius himself speak, as we quote him directly from his original publication:
The mass of a body is a measure of its energy content; if the energy changes by L, the mass changes in the same sense by L/(9*1020) if the energy is measured in ergs and the mass in grams.
You may not recognize this young visionary-looking chad in the photo, but surely you are familiar with this equation. When I first encountered his equation in high school, I just knew it as the “most famous equation in physics”, and slightly later, as my teacher would describe, “the embodiment of Einstein’s Theory of Special Relativity”. Little did I know, a few years later, when I was taking engineering at university, that I would finally come across its deeply erudite connotation.
If you had happened to have been in my class before, and if I had ever said, “the universe is made up of energy and matter (i.e. stuff with mass)”, I’m truly sorry; it was my mistake. You see, I had eventually come to learn that the universe is not “made up of energy and mass”, but the universe is energy! Everything we do and do not experience, or observe, or measure – all manifest themselves in some form of energy. And mass? Well, mass isn’t so much a measure of “the amount of stuff in a material object”, as it is a property which, for our purposes here, can be thought of as the total energies stored within that particular object. Here’s an intuition for you to hold on to: If you so much as breathe, blink, or burp, the energies once stored in you get released to the surrounding typically in the forms of heat, sound, and even light (that is, if you burp really, really hard). As a result, those energies that you gave away made you lighter (though by extremely tiny fractions)! Perhaps, it’s time to eat. Wait, before you do, did I mention that this seemingly simple formula says we cannot travel faster than the speed of light, and therefore you cannot send a therapist or a hitman back to the 1930s, somewhere in Austria, to rewrite history?
#4 : A giant step for physics
Also, did I mention that Equation 2, i.e. Newton’s famous and long-living gravitational equation, does not actually describe all of gravity? In fact, Newton’s perception of the nature of gravity was actually – well, not the whole picture. 250 years later, it would take none other than the genius, Einstein, this time in the midst of World War 1, to come to the rescue once more. In the spring of 2018, I began to really attempt to understand Einstein’s Theory of General Relativity – and truth be told, I just gave up after two weeks or so. But then, when the pandemic hit, in April 2020, I finally decided that I had the space and time (no pun intended) to finally revisit my dusty notebook. Today, I think I have at least somewhat of an intermediate grasp – of the gravitational field equations. You see, the point here is this: very soon, you will come to understand calculus; and know this: no matter what course you decide upon in university later, you can actually extend, perfectly on your own, your knowledge of and technical capacity for calculus to crack what most cannot even begin to perceive – our current best mathematical descriptions of spacetime and thus gravity itself.
Is general relativity hard? The underlying math certainly is, but the key intuition behind all that genius is actually pretty simple. According to GR (which, by the way, had already been rigorously experimented with to a highly precise and broad extent), one cannot perceive space (i.e. the physical, three-dimensional reality we live in), and time (a fourth, non-spatial, temporal dimension), as separate. What has made GR so phenomenal ever since its debut is that it has added so much more nuances to our gravity narrative. To Einstein, the Newtonian framework in which gravity is a universal, invisible, attractive force, had been an incomplete puzzle. Though his depiction isn’t complete, as today’s physics teachers no doubt should be aware of, it is a lot more wholesome, no less significantly more powerful in sheer predictive consistency. But, all you have to know for now is really the intuition: If spacetime can be reduced to an imagined trampoline fabric, put a marble on it, and it creates a small dent in the fabric. Put a golf ball on it, and the dent becomes bigger. Next, put a bowling ball on it, and quickly put it back where it was before Dad starts wondering where it is.
The point is: The heavier an object is (remember: this means bigger mass and thus more total energies), it literally curves the ‘fabric of space and time’, a.k.a. spacetime a.k.a. the trampoline fabric a.k.a. our physical reality. Mind-bending? I unhesitantly and wholeheartedly agree, but stay with me… Now, place the bowling ball, golf ball, and the marble altogether on the trampoline, and align them as if you would the Sun, the Earth, and the Moon, respectively. Finally, watch the analogy play out – notice how the marble (Moon) begins to spiral in- and toward the golf ball (Earth), and how they, together, also begin to do the same with the bowling ball (Sun)? If this experiment is done in zero gravity (plus we need a ‘3-D trampoline’), and as long as time ticks – voila! You’ve just created a miniverse.
Let us now return to the above equation, also known as the Einstein Field Equations (EFE), noting that what we have here is only one way to write it: \ G_{\mu\nu} + \Lambda g_{\mu\nu} = \frac{8\pi G}{c^4} T_{\mu\nu} \ , where:
- \ G_{\mu\nu} \ represents the Einstein tensor, which describes the curvature of spacetime due to gravity,
- \ g_{\mu\nu} \ represents the metric tensor, which describes the geometry of spacetime,
- \Lambda \ represents the cosmological constant, introduced by Einstein to allow for a static universe (but its interpretation has evolved over time, often associated with dark energy and the universe’s expansion),
- \ G \ is the gravitational constant,
- \ c \ is the speed of light, which is notably converts between mass and energy, as in Equation 3 (special relativity),
- \ T_{\mu\nu} \ stands for the energy-momentum tensor, which represents the distribution of matter and energy in spacetime, including information such as density, pressure, and velocity.
The equation essentially states that the curvature of spacetime ��� is related to the energy and momentum content of the universe ���, with the cosmological constant Λ accounting for the energy associated with empty space. The constants � and � govern the overall strength of gravity and the conversion between mass and energy. Viewed as a whole, the left-hand side of the equation represents the geometry of spacetime (i.e. describes how spacetime bends and warps under the influence of gravity), while the right-hand side represents the matter and energy content (i.e. details the distribution and motion of matter and energy that drive this curvature).
Much more importantly, though, beyond this magnificent ‘renaissance of gravity’, both Einstein’s theories of special and general relativity soon sparked a tempest of a revolution, which would go on to define the many hallmark discoveries through the rest of the 20th century – many of which still are greatly impacting our scientific understanding today. Already, other physicists at the time had been contributing and simultaneously debating over the nitty-gritty in the field that had arisen from physics not too long ago – quantum mechanics. Most notably, two of them, one of whom wouldn’t mind imagining a cat’s life-and-death situation, would soon challenge the Einsteinian view at that time, and the very foundation of physics itself.
#5 : The only certainty is that nothing is certain
The most viral thought experiment in physics goes like this: Inside a sealed box, there is a tiny bit of radioactive substance, a vial containing a lethal toxin, and a cat. If the radioactive substance goes through a completely spontaneous process known as decay, it will trigger the vial to release the toxin, and therefore kill the cat. See – the cat’s fate aside – radioactive decay is a natural process, which occurs in the subatomic world. And, for our purposes here, the laws of quantum mechanics dictate that the radioactive substance in the box is going to decay, and not decay at the same time. Wait – what? Does this mean, simultaneously, the cat will die and survive? Now, how do we, the feline lovers, know if the cat actually makes it or not? As far as any observers are concerned, and as many physicists today will tell you – until one of us decides to open the box, the cat will remain, indeed, both dead and alive.
Nobel prize-winning Austrian physicist, Erwin Schrödinger, who presented this twisted humor did so in response to what he thought was the absurdity behind the Copenhagen interpretation of quantum mechanics, which states that a particle can exist in all possible states at the same time, until it is observed. And to understand the weirdness of all of that, a little history helps.
It was 1919. WW1 had just officially ended after the Treaty of Versailles was signed on June 28. But just a month prior to that, a grand experiment would take place, and its results would, in November that same year, get this: confirm Einstein’s Theory of General Relativity. It must have been an incredible moment—not only for Sir Arthur Eddington (and of course, Einstein) who made this astounding achievement possible thanks to a total solar eclipse that year, but also in a much wider reality and beyond the scientific community, it had ushered in a sense of hope after the war.
Five years later, in 1924, French physicist Louis de Broglie published his doctorate thesis, titled “Researches on the quantum theory”, and its central premise would then be proven in 1927. He had utterly transformed the way we think about matter itself, by introducing us to the wave-particle duality, a concept so startlingly hard to accept, much less understand, yet as fundamental to our contemporary quantum knowledge as croissants have been to French culture.
Soon, in the same year as de Broglie’s thesis was confirmed, German theoretical physicist Werner Heisenberg would set the stage for a new era of our quantum theory, by giving us the uncertainty principle. Basically, Equation 5 above says it all. It doesn’t take advanced physics to understand it, really. Here, \Delta x\ refers to a ‘change in position’ (think: How certain do we know where a particle is?), while \Delta p\ refers to a ‘change in momentum’. (This one is hard because you first need an intuition of de Broglie’s duality, but very broadly, you can think: How certain do we know how much energy that particle carries?)
Together, they take on the form of Equation 5, which, if you notice the inequality sign, states that the half of \hbar\ , (known as the reduced Planck’s constant, where \hbar = \frac{h}{2\pi}\ , h being the Planck’s constant, a key number that helps us understand how energy comes in tiny, specific amounts at the smallest level of the world around us), is such a small yet fundamental limit to how precisely we can measure stuff. Consider this: The more we try to pinpoint the location of a particle, the less precisely we’ll be able to know, for instance, how fast it is moving. But, before we actually measure these things, for all we know, the particle can be anywhere and do anything it well pleases! Having a hard time processing all of this? Among many others, Schrödinger and his cat would agree and disagree at the same time.
Past all that scientific rigor, my point is this: Heisenberg’s uncertainty principle is, in a way, a manifestation of our acceptance of the human limit. Look – our physical existence itself prohibits us from fully knowing the very nature of that existence? You frustrated already? So am I. And so was the following guy.
When I hear about Schrödinger's cat, I reach for my gun.
—Stephen Hawking
So, why do you math?
Let’s be honest here: You have to or are at least expected to score well in tests and exams. I understand the pressure, and at some point, you’d probably and perhaps inevitably find yourself not even asking this question anymore. Really, there’s not an easy way to answer it, nor do I think you should actually be bothered by that now, given how excitingly variable your future may be, and the area of work you will eventually find yourself deeply interested in.
In the present, though, and at the very least before we start our lessons, it will help you immensely to understand where you are, and where you are headed, both in respect of your academic needs and personal growth. And in order for me to give you an idea of how you might shed some light on these critical questions right now, let’s borrow from the wisdom of Jordan Ellenberg, professor of mathematics at the University of Wisconsin-Madison, and his aptly-named “four quadrants of the mathematical universe”:
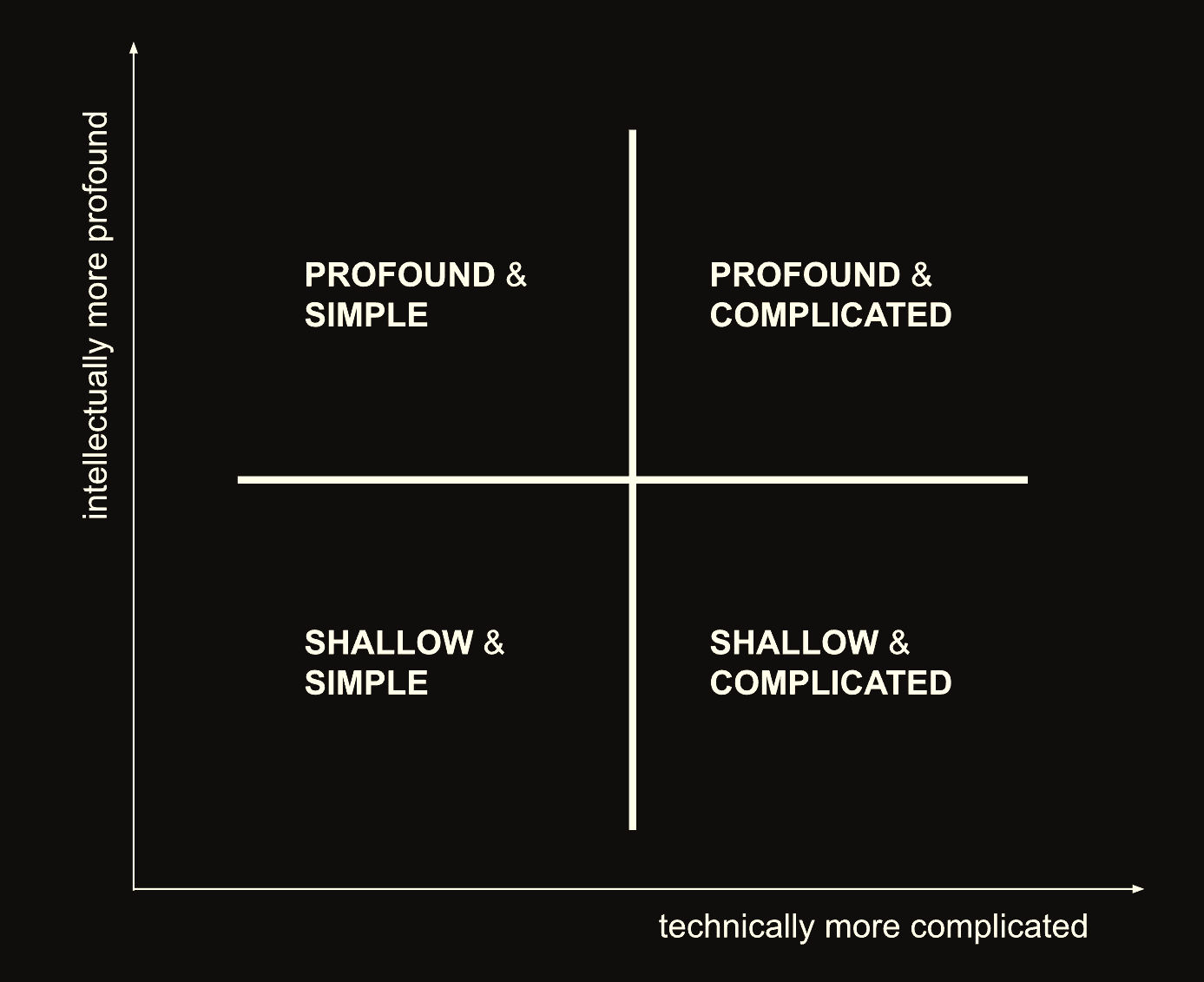
Before we discuss the quadrants, let me explain how you should view the axes. The horizontal axis represents your academic journey. As you grow with time, the theorems, formulas, and reasonings start to extend towards our computing and logical limit as feeble humans. Quite literally, on the other hand, the vertical axis represents – I genuinely think (and wish for more teachers to agree with me) – how meaningful mathematical concepts can turn out to be. It’s easy to relate with me – say, if you have ever wondered: “When will I need to use the quadratic equation?” or “On what occasion would I need to apply the knowledge that the ratio of sine and cosine is tangent?”, just pay attention to the bottom-left quadrant.
Where we are—the shallow and simple
Quadratic equations are shallow and simple. So are linear coordinate geometry, binomial expansion, logarithmic rules, and trigonometric identities. In fact, most of this course we’re about to embark on here is shallow and simple!
It’s a simple trade-off. Before Naruto mastered the Rasengan, I vaguely recall a scene where he had had to first go through the mundane chakra drills and test his abilities on water balloons and rubber balls (to which he certainly ranted a lot to Jiraiya). So, think of IGCSE (or any equivalent O levels) mathematics, as your own genin’s journey in understanding basic chakra dynamics (basic mathematical concepts that make you more conscious about logic), and pushing through chakra drills (exercises and tests that make you more technically fluent). Years before becoming the Hokage, it makes perfect sense to perhaps admit: “I’m too young to know all of what I do, including the reason for why I do so.” Perhaps, with that thinking, Naruto wouldn’t be so grumpy. And you might just feel a lot more comfortable with the idea of uncertainty, and therefore actually more productive in your course of learning.
Where we aspire—the profound and simple
‘Simple’ doesn’t mean ‘unimportant’ – in fact, it’s quite the opposite. What makes simple equations such as \ y=mx+c \ and \ s=r\theta \ important is that they provide you access to the deeper inquiries and revelations that lie beyond them, often in what we teachers like to call ‘applied mathematics’. But I prefer a broader term: reasoning.
It’s easy – and thus tempting – for us to structure a lesson the conventional way. Take geometry for instance; it’s an all-too-common sight where a teacher starts a session and goes, “Today we’ll learn about regular polygons. Each regular polygon has n number of sides, on every vertex shares the same interior as well as exterior angles. To find those angles, let’s look at these formulas…”
Instead, I will start off by telling you that absolutely nothing real in the universe can be accurately measured via representations based on any sort of regular polygons or polyhedrons. The moment we are serious about reality with math and physics and chemistry and biology, things get complicated. That’s why we have regular polygons and polyhedrons. And beyond those, as you get better at the techniques, you get exposed to increasingly complex representations. In other words, you get closer to reality.
Also, just for fun, picture an ant suddenly growing to the size of an adult African elephant; we now know for sure that it will just… die. With only O-level geometry and a little bit of calculus, we can represent this idea with a pretty good approximation, and find out for ourselves at what size the ant will suffocate to its most unfortunate demise. This is because as we increase the surface area by, say, 10 times, we actually increase the volume by 100 times. Of course, this is only if we very roughly represent the ant as a cube. But this principle, known as the square-cube law, is exactly simple and profound. And once you grab an intuition of it, the rest is how skillful you are at going from an elephant-sized ant to predicting the death of a black hole.
Where we struggle—the shallow and complicated
Say, you want to understand quantum mechanics, and the conflicts that our best theories currently pose against our best interpretation of gravity (i.e. general relativity), you have no choice but to push your technical limits beyond what high schools are designed to provide. You have to at least grind through electrodynamics (for quantum mechanics) and tensor calculus (for GR). They are incredibly difficult. But once you go past all that, you reach the top-right quadrant – kind of like the frontline, if our quest for knowledge were a battle.
Where greatness lies—the profound and complicated
You may not know the equations and proofs, or comprehend any of the reasons behind them, but you know the names. Like Muhammad Ali and Bruce Lee are to the world of martial arts, giants whose names I simply cannot fit in a paragraph going from Archimedes to Feynman had not only been in the top-right quadrant; they were responsible for creating, extending, and challenging its contents and confines.
Yes, the top-right quadrant is not for the faint-hearted, but also in this modern era, who says you can’t or won’t be there? If you want, I say start from the two quadrants on the left, i.e. where we are, and where we aspire to be, over our Oxbridge Mathematics course. Think big, act small.
To be continued...
Thanks for reading! This is the first part of a trilogy that aims at making math accessible to readers from all walks of life. Stay tuned for Part 2.
Let's talk!
TRIAL LESSON ONLY AT 49 MYR
Read our latest.
Access learning resources.
COMING SOON
